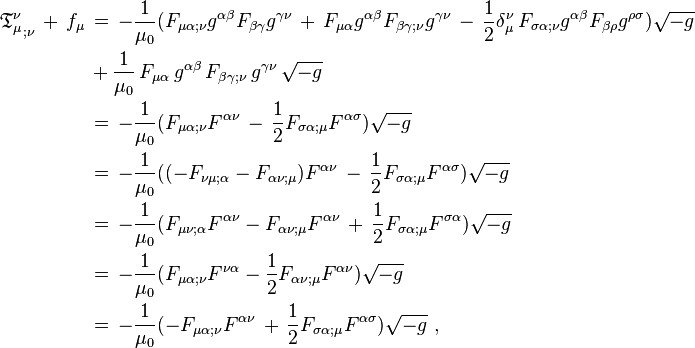Electromagnetic Stress-energy Tensor
As part of the source term in the Einstein field equations, the electromagnetic stress-energy tensor is a covariant symmmetric tensor
which is trace-free
because electromagnetism propagates at the invariant speed.
In the expression for the conservation of energy and linear momentum, the electromagnetic stress-energy tensor is best represented as a mixed tensor density
From the equations above, one can show that
where the semicolon indicates a covariant derivative.
This can be rewritten as
which says that the decrease in the electromagnetic energy is the same as the work done by the electromagnetic field on the gravitational field plus the work done on matter (via the Lorentz force), and similarly the rate of decrease in the electromagnetic linear momentum is the electromagnetic force exerted on the gravitational field plus the Lorentz force exerted on matter.
Derivation of conservation law
which is zero because it is the negative of itself (see four lines above).
Read more about this topic: Maxwell's Equations In Curved Spacetime