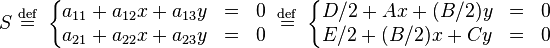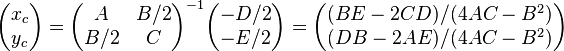Matrix Representation of Conic Sections - Center

Center

In the center of the conic, the gradient of the quadratic form Q vanishes, so: 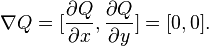\nabla Q = = .

We can calculate the center by taking the first two rows of the associated matrix, multiplying each by (x, y, 1)T, setting both inner products equal to 0, and solving the system.

S \ \stackrel{\mathrm{def}}{=}\ \left\{ \begin{matrix} a_{11} + a_{12}x + a_{13}y & = & 0 \\ a_{21} + a_{22}x + a_{23}y & = & 0 \end{matrix} \right. \ \stackrel{\mathrm{def}}{=}\ \left\{\begin{matrix} D/2 + Ax + (B/2)y & = & 0 \\ E/2 + (B/2)x + Cy & = & 0 \end{matrix} \right.

This becomes

\begin{pmatrix} x_c \\ y_c \end{pmatrix} = \begin{pmatrix} A & B/2 \\ B/2 & C \end{pmatrix}^{-1} \begin{pmatrix} -D/2 \\ -E/2 \end{pmatrix} = \begin{pmatrix} (BE-2CD)/(4AC-B^2) \\ (DB-2AE)/(4AC-B^2) \end{pmatrix}

Note that in the case of a parabola, defined by (4AC-B2) = 0, there is no center since the above denominators become zero.

Read more about this topic:  Matrix Representation Of Conic Sections

Famous quotes containing the word center:

    “Whether talking about addiction, taxation [on cigarettes] or education [about smoking], there is always at the center of the conversation an essential conundrum: How come we’re selling this deadly stuff anyway?”
    —Anna Quindlen (b. 1952)

    “I am the center of the world, but the control panel seems to be somewhere else.”
    —Mason Cooley (b. 1927)

    “When the landscape buckles and jerks around, when a dust column of debris rises from the collapse of a block of buildings on bodies that could have been your own, when the staves of history fall awry and the barrel of time bursts apart, some turn to prayer, some to poetry: words in the memory, a stained book carried close to the body, the notebook scribbled by hand—a center of gravity.”
    —Adrienne Rich (b. 1929)