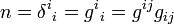List of Formulas in Riemannian Geometry - Christoffel Symbols, Covariant Derivative

Christoffel Symbols, Covariant Derivative

In a smooth coordinate chart, the Christoffel symbols of the first kind are given by

$\Gamma_{kij}=\frac12 \left( \frac{\partial}{\partial x^j} g_{ki} +\frac{\partial}{\partial x^i} g_{kj} -\frac{\partial}{\partial x^k} g_{ij} \right) =\frac12 \left( g_{ki,j} + g_{kj,i} - g_{ij,k} \right) \,,$

and the Christoffel symbols of the second kind by

$\begin{align} \Gamma^m{}_{ij} &= g^{mk}\Gamma_{kij}\\ &=\frac12\, g^{mk} \left( \frac{\partial}{\partial x^j} g_{ki} +\frac{\partial}{\partial x^i} g_{kj} -\frac{\partial}{\partial x^k} g_{ij} \right) =\frac12\, g^{mk} \left( g_{ki,j} + g_{kj,i} - g_{ij,k} \right) \,. \end{align}$

Here is the inverse matrix to the metric tensor . In other words,

$\delta^i{}_j = g^{ik}g_{kj}$

and thus

$n = \delta^i{}_i = g^i{}_i = g^{ij}g_{ij}$

is the dimension of the manifold.

Christoffel symbols satisfy the symmetry relation

$\Gamma^i{}_{jk}=\Gamma^i{}_{kj} \,,$

which is equivalent to the torsion-freeness of the Levi-Civita connection.

The contracting relations on the Christoffel symbols are given by

and

where |g| is the absolute value of the determinant of the metric tensor . These are useful when dealing with divergences and Laplacians (see below).

The covariant derivative of a vector field with components is given by:

$v^i {}_{;j}=\nabla_j v^i=\frac{\partial v^i}{\partial x^j}+\Gamma^i{}_{jk}v^k$

and similarly the covariant derivative of a -tensor field with components is given by:

$v_{i;j}=\nabla_j v_i=\frac{\partial v_i}{\partial x^j}-\Gamma^k{}_{ij} v_k$

For a -tensor field with components this becomes

$v^{ij}{}_{;k}=\nabla_k v^{ij}=\frac{\partial v^{ij}}{\partial x^k} +\Gamma^i{}_{k\ell}v^{\ell j}+\Gamma^j{}_{k\ell}v^{i\ell}$

and likewise for tensors with more indices.

The covariant derivative of a function (scalar) is just its usual differential:

$\nabla_i \phi=\phi_{;i}=\phi_{,i}=\frac{\partial \phi}{\partial x^i}$

Because the Levi-Civita connection is metric-compatible, the covariant derivatives of metrics vanish,

$\nabla_k g_{ij} = \nabla_k g^{ij} = 0$

The geodesic starting at the origin with initial speed has Taylor expansion in the chart:

$X(t)^i=tv^i-\frac{t^2}{2}\Gamma^i{}_{jk}v^jv^k+O(t^2)$

Read more about this topic: List Of Formulas In Riemannian Geometry

Famous quotes containing the word derivative:

“Poor John Field!—I trust he does not read this, unless he will improve by it,—thinking to live by some derivative old-country mode in this primitive new country.... With his horizon all his own, yet he a poor man, born to be poor, with his inherited Irish poverty or poor life, his Adam’s grandmother and boggy ways, not to rise in this world, he nor his posterity, till their wading webbed bog-trotting feet get talaria to their heels.”
—Henry David Thoreau (1817–1862)