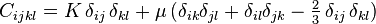Linear Elasticity - Isotropic Homogeneous Media

Isotropic Homogeneous Media

In isotropic media, the stiffness tensor gives the relationship between the stresses (resulting internal stresses) and the strains (resulting deformations). For an isotropic medium, the stiffness tensor has no preferred direction: an applied force will give the same displacements (relative to the direction of the force) no matter the direction in which the force is applied. In the isotropic case, the stiffness tensor may be written:

$C_{ijkl} = K \, \delta_{ij}\, \delta_{kl} +\mu\, (\delta_{ik}\delta_{jl}+\delta_{il}\delta_{jk}-\textstyle{\frac{2}{3}}\, \delta_{ij}\,\delta_{kl}) \,\!$

where is the Kronecker delta, K is the bulk modulus (or incompressibility), and is the shear modulus (or rigidity), two elastic moduli. If the medium is inhomogeneous, the isotropic model is sensible if either the medium is piecewise-constant or weakly inhomogeneous; in the strongly inhomogeneous smooth model, anisotropy has to be accounted for. If the medium is homogeneous, then the elastic moduli will not be a function of position in the medium. The constitutive equation may now be written as:

$\sigma_{ij} =K\delta_{ij}\varepsilon_{kk}+2\mu(\varepsilon_{ij}-\textstyle{\frac{1}{3}}\delta_{ij}\varepsilon_{kk}). \,\!$

This expression separates the stress into a scalar part on the left which may be associated with a scalar pressure, and a traceless part on the right which may be associated with shear forces. A simpler expression is:

$\sigma_{ij} =\lambda \delta_{ij} \varepsilon_{kk}+2\mu\varepsilon_{ij} \,\!$

where λ is Lamé's first parameter. Since the constitutive equation is simply a set of linear equations, the strain may be expressed as a function of the stresses as:

$\varepsilon_{ij} = \frac{1}{9K}\delta_{ij}\sigma_{kk} + \frac{1}{2\mu}\left(\sigma_{ij}-\textstyle{\frac{1}{3}}\delta_{ij}\sigma_{kk}\right) \,\!$

which is again, a scalar part on the left and a traceless shear part on the right. More simply:

$\varepsilon_{ij} =\frac{1}{2\mu}\sigma_{ij}-\frac{\nu}{E}\delta_{ij}\sigma_{kk}=\frac{1}{E} \,\!$

where ν is Poisson's ratio and E is Young's modulus.

Read more about this topic: Linear Elasticity

Famous quotes containing the words homogeneous and/or media:

“O my Brothers! love your Country. Our Country is our home, the home which God has given us, placing therein a numerous family which we love and are loved by, and with which we have a more intimate and quicker communion of feeling and thought than with others; a family which by its concentration upon a given spot, and by the homogeneous nature of its elements, is destined for a special kind of activity.”
—Giuseppe Mazzini (1805–1872)

“The media no longer ask those who know something ... to share that knowledge with the public. Instead they ask those who know nothing to represent the ignorance of the public and, in so doing, to legitimate it.”
—Serge Daney (1944–1992)