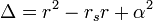Kerr Metric - Mathematical Form

Mathematical Form

The Kerr metric describes the geometry of spacetime in the vicinity of a mass M rotating with angular momentum J

c^{2} d\tau^{2} = \left( 1 - \frac{r_{s} r}{\rho^{2}} \right) c^{2} dt^{2} - \frac{\rho^{2}}{\Delta} dr^{2} - \rho^{2} d\theta^{2} -
\left( r^{2} + \alpha^{2} + \frac{r_{s} r \alpha^{2}}{\rho^{2}} \sin^{2} \theta \right) \sin^{2} \theta \ d\phi^{2} + \frac{2r_{s} r\alpha \sin^{2} \theta }{\rho^{2}} \, c \, dt \, d\phi

where the coordinates are standard spherical coordinate system, and rs is the Schwarzschild radius

r_{s} = \frac{2GM}{c^{2}}

and where the length-scales α, ρ and Δ have been introduced for brevity

\alpha = \frac{J}{Mc}
\ \rho^{2} = r^{2} + \alpha^{2} \cos^{2} \theta
\ \Delta = r^{2} - r_{s} r + \alpha^{2}

In the non-relativistic limit where M (or, equivalently, rs) goes to zero, the Kerr metric becomes the orthogonal metric for the oblate spheroidal coordinates

c^{2} d\tau^{2} = c^{2} dt^{2} - \frac{\rho^{2}}{r^{2} + \alpha^{2}} dr^{2} - \rho^{2} d\theta^{2} - \left( r^{2} + \alpha^{2} \right) \sin^{2}\theta d\phi^{2}

which are equivalent to the Boyer-Lindquist coordinates

Read more about this topic:  Kerr Metric

Famous quotes containing the words mathematical form, mathematical and/or form:

    “The most distinct and beautiful statement of any truth must take at last the mathematical form.”
    —Henry David Thoreau (1817–1862)

    “As we speak of poetical beauty, so ought we to speak of mathematical beauty and medical beauty. But we do not do so; and that reason is that we know well what is the object of mathematics, and that it consists in proofs, and what is the object of medicine, and that it consists in healing. But we do not know in what grace consists, which is the object of poetry.”
    —Blaise Pascal (1623–1662)

    “I do not believe in democracy, but I am perfectly willing to admit that it provides the only really amusing form of government ever endured by mankind.”
    —H.L. (Henry Lewis)