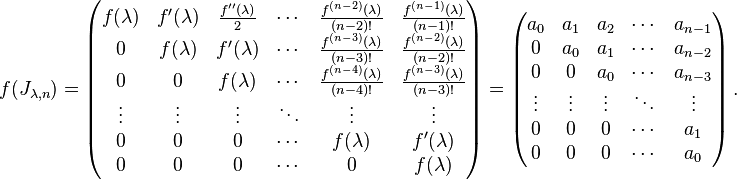Jordan Matrix - Functions of Matrices

Functions of Matrices

Let (i.e. a complex matrix) and be the change of basis matrix to the Jordan normal form of, i.e. . Now let be a holomorphic function on an open set such that, i.e. the spectrum of the matrix is contained inside the domain of holomorphy of . Let

be the power series expansion of around, which will be hereinafter supposed to be 0 for simplicity's sake. The matrix is then defined via the following formal power series

is absolutely convergent respect to the Euclidean norm of . To put it in another way, converges absolutely for every square matrix whose spectral radius is less than the radius of convergence of around and is uniformly convergent on any compact subsets of satisfying this property in the matrix Lie group topology.

The Jordan normal form allows the computation of functions of matrices without explicitly computing an infinite series, which is one of the main achievements of Jordan matrices. Using the facts that the power of a diagonal block matrix is the diagonal block matrix whose blocks are the powers of the respective blocks, i.e., and that, the above matrix power series becomes

where the last series must not be computed explicitly via power series of every Jordan block. In fact, if, any holomorphic function of a Jordan block is the following upper triangular matrix:

As a consequence of this, the computation of any functions of a matrix is straightforward whenever its Jordan normal form and its change-of-basis matrix are known. Also, i.e. every eigenvalue corresponds to the eigenvalue, but it has, in general, different algebraic multiplicity, geometric multiplicity and index. However, the algebraic multiplicity may be computed as follows:

The function of a linear transformation between vector spaces can be defined in a similar way according to the holomorphic functional calculus, where Banach space and Riemann surface theories play a fundamental role. Anyway, in the case of finite-dimensional spaces, both theories perfectly match.