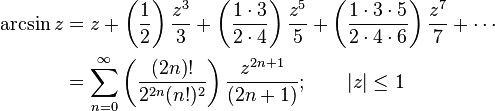Inverse Trigonometric Functions - Infinite Series

Infinite Series

Like the sine and cosine functions, the inverse trigonometric functions can be calculated using infinite series, as follows:

\begin{align} \arcsin z & {}= z + \left( \frac {1} {2} \right) \frac {z^3} {3} + \left( \frac {1 \cdot 3} {2 \cdot 4} \right) \frac {z^5} {5} + \left( \frac{1 \cdot 3 \cdot 5} {2 \cdot 4 \cdot 6 } \right) \frac{z^7} {7} + \cdots\\ & {}= \sum_{n=0}^\infty \left( \frac {(2n)!} {2^{2n}(n!)^2} \right) \frac {z^{2n+1}} {(2n+1)} ; \qquad | z | \le 1 \end{align}


\begin{align} \arccos z & {}= \frac {\pi} {2} - \arcsin z \\ & {}= \frac {\pi} {2} - (z + \left( \frac {1} {2} \right) \frac {z^3} {3} + \left( \frac {1 \cdot 3} {2 \cdot 4} \right) \frac {z^5} {5} + \left( \frac{1 \cdot 3 \cdot 5} {2 \cdot 4 \cdot 6 } \right) \frac{z^7} {7} + \cdots ) \\ & {}= \frac {\pi} {2} - \sum_{n=0}^\infty \left( \frac {(2n)!} {2^{2n}(n!)^2} \right) \frac {z^{2n+1}} {(2n+1)} ; \qquad | z | \le 1 \end{align}


\begin{align} \arctan z & {}= z - \frac {z^3} {3} +\frac {z^5} {5} -\frac {z^7} {7} +\cdots \\ & {}= \sum_{n=0}^\infty \frac {(-1)^n z^{2n+1}} {2n+1} ; \qquad | z | \le 1 \qquad z \neq i,-i \end{align}


\begin{align} \arccot z & {}= \frac {\pi} {2} - \arctan z \\ & {}= \frac {\pi} {2} - ( z - \frac {z^3} {3} +\frac {z^5} {5} -\frac {z^7} {7} +\cdots ) \\ & {}= \frac {\pi} {2} - \sum_{n=0}^\infty \frac {(-1)^n z^{2n+1}} {2n+1} ; \qquad | z | \le 1 \qquad z \neq i,-i \end{align}


\begin{align} \arcsec z & {}= \arccos {(1/z)} \\ & {}= \frac {\pi} {2} - (z^{-1} + \left( \frac {1} {2} \right) \frac {z^{-3}} {3} + \left( \frac {1 \cdot 3} {2 \cdot 4} \right) \frac {z^{-5}} {5} + \left( \frac{1 \cdot 3 \cdot 5} {2 \cdot 4 \cdot 6 } \right) \frac{z^{-7}} {7} + \cdots ) \\ & {}= \frac {\pi} {2} - \sum_{n=0}^\infty \left( \frac {(2n)!} {2^{2n}(n!)^2} \right) \frac {z^{-(2n+1)}} {(2n+1)} ; \qquad \left| z \right| \ge 1 \end{align}


\begin{align} \arccsc z & {}= \arcsin {(1/z)} \\ & {}= z^{-1} + \left( \frac {1} {2} \right) \frac {z^{-3}} {3} + \left( \frac {1 \cdot 3} {2 \cdot 4 } \right) \frac {z^{-5}} {5} + \left( \frac {1 \cdot 3 \cdot 5} {2 \cdot 4 \cdot 6} \right) \frac {z^{-7}} {7} +\cdots \\ & {}= \sum_{n=0}^\infty \left( \frac {(2n)!} {2^{2n}(n!)^2} \right) \frac {z^{-(2n+1)}} {2n+1} ; \qquad \left| z \right| \ge 1 \end{align}


Leonhard Euler found a more efficient series for the arctangent, which is:

(Notice that the term in the sum for n= 0 is the empty product which is 1.)


Alternatively, this can be expressed:

Read more about this topic:  Inverse Trigonometric Functions

Famous quotes containing the words infinite and/or series:

    “Alas, poor Yorick! I knew him, Horatio: a fellow of infinite jest, of most excellent fancy.... Where be your jibes now, your gambols, your songs, your flashes of merriment that were wont to set the table on a roar?”
    —William Shakespeare (1564–1616)

    “There is in every either-or a certain naivete which may well befit the evaluator, but ill- becomes the thinker, for whom opposites dissolve in series of transitions.”
    —Robert Musil (1880–1942)