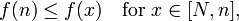Integral Test For Convergence - Proof

Proof

The proof basically uses the comparison test, comparing the term f(n) with the integral of f over the intervals and, respectively.

Since f is a monotone decreasing function, we know that

f(x)\le f(n)\quad\text{for }x\in[n,\infty)

and

f(n)\le f(x)\quad\text{for }x\in,

hence for every n larger than N

\int_n^{n+1} f(x)\,dx \le\int_{n}^{n+1} f(n)\,dx =f(n) =\int_{n-1}^{n} f(n)\,dx \le\int_{n-1}^n f(x)\,dx.

Since the lower estimate is also valid for f(N), we get by summation over all n from N to some larger integer M

\int_N^{M+1}f(x)\,dx\le\sum_{n=N}^Mf(n)\le f(N)+\int_N^M f(x)\,dx.

Regarding the last two terms of the inequality: for every (in the summed series), a (in the integral term) is greater than the former term. Working backward from (the final term in the summation), there is an integral increment that is greater than its summation term, except for (because the domain is bounded by N), which is then added to the integral side of the inequality. Thus,

Letting M tend to infinity, the result follows.

Read more about this topic:  Integral Test For Convergence

Famous quotes containing the word proof:

    “It comes to pass oft that a terrible oath, with a swaggering accent sharply twanged off, gives manhood more approbation than ever proof itself would have earned him.”
    —William Shakespeare (1564–1616)

    “If any doubt has arisen as to me, my country [Virginia] will have my political creed in the form of a “Declaration &c.” which I was lately directed to draw. This will give decisive proof that my own sentiment concurred with the vote they instructed us to give.”
    —Thomas Jefferson (1743–1826)

    “The moment a man begins to talk about technique that’s proof that he is fresh out of ideas.”
    —Raymond Chandler (1888–1959)