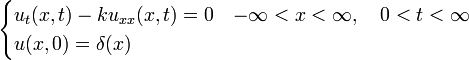Heat Equation - Fundamental Solutions

Fundamental Solutions

A fundamental solution, also called a heat kernel, is a solution of the heat equation corresponding to the initial condition of an initial point source of heat at a known position. These can be used to find a general solution of the heat equation over certain domains; see, for instance, (Evans 1998) for an introductory treatment.

In one variable, the Green's function is a solution of the initial value problem

\begin{cases} u_t(x,t) - k u_{xx}(x,t) = 0& -\infty<x<\infty,\quad 0<t<\infty\\ u(x,0)=\delta(x)& \end{cases}

where δ is the Dirac delta function. The solution to this problem is the fundamental solution

One can obtain the general solution of the one variable heat equation with initial condition u(x, 0) = g(x) for -∞ < x < ∞ and 0 < t < ∞ by applying a convolution:

In several spatial variables, the fundamental solution solves the analogous problem

\begin{cases} u_t(\mathbf{x},t) - k \sum_{i=1}^nu_{x_ix_i}(\mathbf{x},t) = 0\\ u(\mathbf{x},0)=\delta(\mathbf{x}) \end{cases}

in -∞ < x i < ∞, i = 1,...,n, and 0 < t < ∞. The n-variable fundamental solution is the product of the fundamental solutions in each variable; i.e.,

The general solution of the heat equation on Rn is then obtained by a convolution, so that to solve the initial value problem with u(x, t = 0) = g(x), one has

The general problem on a domain Ω in Rn is

\begin{cases} u_t(\mathbf{x},t) - k \sum_{i=1}^nu_{x_ix_i}(\mathbf{x},t) = 0& \mathbf{x}\in\Omega\quad 0<t<\infty\\ u(\mathbf{x},0)=g(\mathbf{x})&\mathbf{x}\in\Omega \end{cases}

with either Dirichlet or Neumann boundary data. A Green's function always exists, but unless the domain Ω can be readily decomposed into one-variable problems (see below), it may not be possible to write it down explicitly. The method of images provides one additional technique for obtaining Green's functions for non-trivial domains.

Read more about this topic:  Heat Equation

Famous quotes containing the words fundamental and/or solutions:

    “In this choice of inheritance we have given to our frame of polity the image of a relation in blood; binding up the constitution of our country with our dearest domestic ties; adopting our fundamental laws into the bosom of our family affections; keeping inseparable and cherishing with the warmth of all their combined and mutually reflected charities, our state, our hearths, our sepulchres, and our altars.”
    —Edmund Burke (1729–1797)

    “The anorexic prefigures this culture in rather a poetic fashion by trying to keep it at bay. He refuses lack. He says: I lack nothing, therefore I shall not eat. With the overweight person, it is the opposite: he refuses fullness, repletion. He says, I lack everything, so I will eat anything at all. The anorexic staves off lack by emptiness, the overweight person staves off fullness by excess. Both are homeopathic final solutions, solutions by extermination.”
    —Jean Baudrillard (b. 1929)