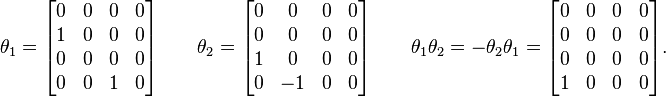Grassmann Number - Matrix Representations

Matrix Representations

Grassmann numbers can always be represented by matrices. Consider, for example, the Grassmann algebra generated by two Grassmann numbers and . These Grassmann numbers can be represented by 4×4 matrices:

$\theta_1 = \begin{bmatrix} 0 & 0 & 0 & 0\\ 1 & 0 & 0 & 0\\ 0 & 0 & 0 & 0\\ 0 & 0 & 1 & 0\\ \end{bmatrix}\qquad \theta_2 = \begin{bmatrix} 0&0&0&0\\ 0&0&0&0\\ 1&0&0&0\\ 0&-1&0&0\\ \end{bmatrix}\qquad \theta_1\theta_2 = -\theta_2\theta_1 = \begin{bmatrix} 0&0&0&0\\ 0&0&0&0\\ 0&0&0&0\\ 1&0&0&0\\ \end{bmatrix}.$

In general, a Grassmann algebra on n generators can be represented by 2n × 2n square matrices. Physically, these matrices can be thought of as raising operators acting on a Hilbert space of n identical fermions in the occupation number basis. Since the occupation number for each fermion is 0 or 1, there are 2n possible basis states. Mathematically, these matrices can be interpreted as the linear operators corresponding to left exterior multiplication on the Grassmann algebra itself.

Read more about this topic: Grassmann Number

Famous quotes containing the word matrix:

““The matrix is God?”
“In a manner of speaking, although it would be more accurate ... to say that the matrix has a God, since this being’s omniscience and omnipotence are assumed to be limited to the matrix.”
“If it has limits, it isn’t omnipotent.”
“Exactly.... Cyberspace exists, insofar as it can be said to exist, by virtue of human agency.””
—William Gibson (b. 1948)