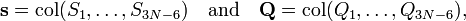GF Method - The GF Method

The GF Method

A non-linear molecule consisting of N atoms has 3N-6 internal degrees of freedom, because positioning a molecule in three-dimensional space requires three degrees of freedom and the description of its orientation in space requires another three degree of freedom. These degrees of freedom must be subtracted from the 3N degrees of freedom of a system of N particles.

The atoms in a molecule are bound by a potential energy surface (PES) (or a force field) which is a function of 3N-6 coordinates. The internal degrees of freedom q₁, ..., q_3N-6 describing the PES in an optimum way are often non-linear; they are for instance valence coordinates, such as bending and torsion angles and bond stretches. It is possible to write the quantum mechanical kinetic energy operator for such curvilinear coordinates, but it is hard to formulate a general theory applicable to any molecule. This is why Wilson linearized the internal coordinates by assuming small displacements. The linearized version of the internal coordinate q_t is denoted by S_t.

The PES V can be Taylor expanded around its minimum in terms of the S_t. The third term (the Hessian of V) evaluated in the minimum is a force derivative matrix F. In the harmonic approximation the Taylor series is ended after this term. The second term, containing first derivatives, is zero because it is evaluated in the minimum of V. The first term can be included in the zero of energy. Thus,

The classical vibrational kinetic energy has the form:

where g_st is an element of the metric tensor of the internal (curvilinear) coordinates. The dots indicate time derivatives. Mixed terms generally present in curvilinear coordinates are not present here, because only linear coordinate transformations are used. Evaluation of the metric tensor g in the minimum q0 of V gives the positive definite and symmetric matrix G = g(q0)−1. One can solve the following two matrix problems simultaneously

$\mathbf{L}^\mathrm{T} \mathbf{F} \mathbf{L} =\boldsymbol{\Phi} \quad \mathrm{and}\quad \mathbf{L}^\mathrm{T} \mathbf{G}^{-1} \mathbf{L} = \mathbf{E},$

since they are equivalent to the generalized eigenvalue problem

$\mathbf{G} \mathbf{F} \mathbf{L} = \mathbf{L} \boldsymbol{\Phi},$

where where f_i is equal to ( is the frequency of normal mode i); is the unit matrix. The matrix L−1 contains the normal coordinates Q_k in its rows:

Because of the form of the generalized eigenvalue problem, the method is called the GF method, often with the name of its originator attached to it: Wilson's GF method. By matrix transposition in both sides of the equation and using the fact that both G and F are symmetric matrices, as are diagonal matrices, one can recast this equation into a very similar one for FG . This is why the method is also referred to as Wilson's FG method.

We introduce the vectors

$\mathbf{s} = \operatorname{col}(S_1,\ldots, S_{3N-6}) \quad\mathrm{and}\quad \mathbf{Q} = \operatorname{col}(Q_1,\ldots, Q_{3N-6}),$

which satisfy the relation

$\mathbf{s} = \mathbf{L} \mathbf{Q}.$

Upon use of the results of the generalized eigenvalue equation, the energy E = T + V (in the harmonic approximation) of the molecule becomes,

$2E = \dot{\mathbf{s}}^\mathrm{T} \mathbf{G}^{-1}\dot{\mathbf{s}}+ \mathbf{s}^\mathrm{T}\mathbf{F}\mathbf{s}$

$= \dot{\mathbf{Q}}^\mathrm{T} \; \left( \mathbf{L}^\mathrm{T} \mathbf{G}^{-1} \mathbf{L}\right) \; \dot{\mathbf{Q}}+ \mathbf{Q}^\mathrm{T} \left( \mathbf{L}^\mathrm{T}\mathbf{F}\mathbf{L}\right)\; \mathbf{Q}$

$= \dot{\mathbf{Q}}^\mathrm{T}\dot{\mathbf{Q}} + \mathbf{Q}^\mathrm{T}\boldsymbol{\Phi}\mathbf{Q} = \sum_{t=1}^{3N-6} \big( \dot{Q}_t^2 + f_t Q_t^2 \big).$

The Lagrangian L = T - V is

$L = \frac{1}{2} \sum_{t=1}^{3N-6} \big( \dot{Q}_t^2 - f_t Q_t^2 \big).$

The corresponding Lagrange equations are identical to the Newton equations

$\ddot{Q}_t + f_t \,Q_t = 0$

for a set of uncoupled harmonic oscillators. These ordinary second-order differential equations are easily solved, yielding Q_t as a function of time; see the article on harmonic oscillators.

Famous quotes containing the word method:

“I know no method to secure the repeal of bad or obnoxious laws so effective as their stringent execution.”
—Ulysses S. Grant (1822–1885)