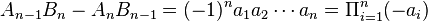Fundamental Recurrence Formulas - The Determinant Formula

The Determinant Formula

It can be shown, by induction, that the determinant formula

$A_{n-1}B_n - A_nB_{n-1} = (-1)^na_1a_2\cdots a_n = \Pi_{i=1}^n (-a_i)\,$

holds for all positive integers n > 0. If neither B_n-1 nor B_n is zero, this relationship can also be used to express the difference between two successive convergents of the continued fraction.

$x_{n-1} - x_n = \frac{A_{n-1}}{B_{n-1}} - \frac{A_n}{B_n} = (-1)^n \frac{a_1a_2\cdots a_n}{B_nB_{n-1}} = \frac{\Pi_{i=1}^n (-a_i)}{B_nB_{n-1}}\,$

It is necessary but not sufficient for the convergence of an infinite continued fraction that the difference between successive convergents approaches zero; this is the subject of the convergence problem. (Note: By definition, the continued fraction converges if the sequence of convergents has a limit.)

Read more about this topic: Fundamental Recurrence Formulas

Famous quotes containing the word formula:

“The formula for achieving a successful relationship is simple: you should treat all disasters as if they were trivialities but never treat a triviality as if it were a disaster.”
—Quentin Crisp (b. 1908)