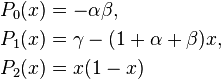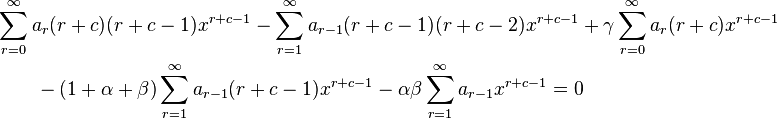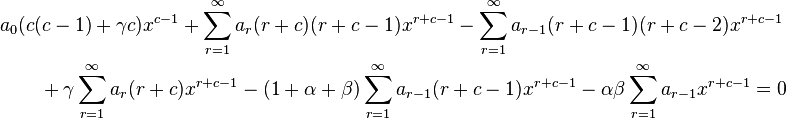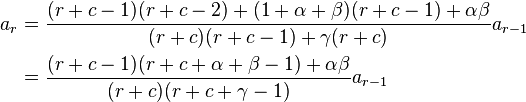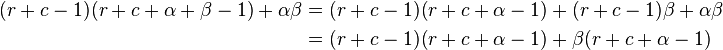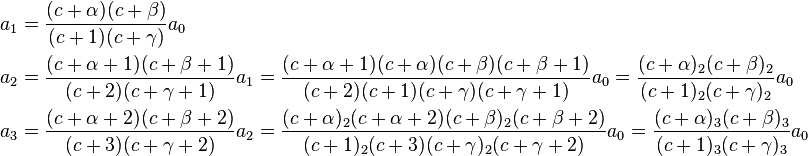Frobenius Solution To The Hypergeometric Equation - Solution Around x = 0

Solution Around x = 0

Let

\begin{align} P_0(x) &= -\alpha \beta, \\ P_1(x) &= \gamma - (1+\alpha +\beta )x, \\ P_2(x) &= x(1-x) \end{align}

Then

Hence, x = 0 and x = 1 are singular points. Let's start with x = 0. To see if it is regular, we study the following limits:

\begin{align} \lim_{x \to a} \frac{(x - a) P_1(x)}{P_2(x)} &=\lim_{x \to 0} \frac{(x - 0)(\gamma - (1 + \alpha + \beta)x)}{x(1 - x)}=\lim_{x \to 0} \frac{x(\gamma - (1 + \alpha + \beta)x)}{x(1 - x)}= \gamma \\ \lim_{x \to a} \frac{(x - a)^2 P_0(x)}{P_2(x)} &= \lim_{x \to 0} \frac{(x - 0)^2(-\alpha \beta)}{x(1 - x)} = \lim_{x \to 0} \frac{x^2 (-\alpha \beta)}{x(1 - x)} = 0 \end{align}

Hence, both limits exist and x = 0 is a regular singular point. Therefore, we assume the solution takes the form

with a0 ≠ 0. Hence,

\begin{align} y' &= \sum_{r = 0}^\infty a_r(r + c) x^{r + c - 1} \\ y'' &= \sum_{r = 0}^\infty a_r(r + c)(r + c - 1) x^{r + c - 2}. \end{align}

Substituting these into the hypergeometric equation, we get

That is,

In order to simplify this equation, we need all powers to be the same, equal to r + c − 1, the smallest power. Hence, we switch the indices as follows:

\begin{align} &\sum_{r = 0}^\infty a_r(r + c)(r + c - 1)x^{r + c - 1} -\sum_{r = 1}^\infty a_{r - 1}(r + c - 1)(r + c - 2) x^{r + c - 1} +\gamma \sum_{r = 0}^\infty a_r(r + c) x^{r + c - 1} \\ &\qquad -(1 + \alpha + \beta) \sum_{r = 1}^\infty a_{r - 1}(r + c - 1) x^{r + c - 1}-\alpha \beta \sum_{r = 1}^\infty a_{r - 1} x^{r + c - 1} =0 \end{align}

Thus, isolating the first term of the sums starting from 0 we get

\begin{align} &a_0 (c(c-1) + \gamma c) x^{c - 1}+ \sum_{r = 1}^\infty a_r(r + c)(r + c - 1) x^{r + c - 1} -\sum_{r = 1}^\infty a_{r - 1}(r + c - 1)(r + c - 2) x^{r + c - 1} \\ &\qquad + \gamma \sum_{r = 1}^\infty a_r(r + c) x^{r + c - 1}-(1 + \alpha + \beta) \sum_{r = 1}^\infty a_{r - 1}(r + c - 1) x^{r + c - 1}-\alpha \beta \sum_{r = 1}^\infty a_{r - 1} x^{r + c - 1}= 0 \end{align}

Now, from the linear independence of all powers of x, that is, of the functions 1, x, x2, etc., the coefficients of xk vanish for all k. Hence, from the first term, we have

which is the indicial equation. Since a0 ≠ 0, we have

Hence,

Also, from the rest of the terms, we have

Hence,

\begin{align} a_r &= \frac{(r + c - 1)(r + c - 2) + (1 + \alpha + \beta)(r + c - 1) + \alpha\beta} {(r + c)(r + c - 1) + \gamma(r + c)} a_{r - 1} \\ &= \frac{(r + c -1)(r + c + \alpha + \beta - 1) + \alpha\beta}{(r + c)(r + c + \gamma - 1)} a_{r - 1} \end{align}

But

\begin{align} (r + c - 1)(r + c + \alpha + \beta - 1) + \alpha\beta &= (r + c - 1)(r + c + \alpha - 1) + (r + c - 1)\beta + \alpha\beta \\ &= (r + c - 1)(r + c + \alpha - 1) + \beta(r + c + \alpha - 1) \end{align}

Hence, we get the recurrence relation

Let's now simplify this relation by giving ar in terms of a0 instead of ar−1. From the recurrence relation (note: below, expressions of the form (u)r refer to the Pochhammer symbol).

\begin{align} a_1 &= \frac{(c + \alpha)(c + \beta)}{(c + 1)(c + \gamma)} a_0 \\ a_2 &= \frac{(c + \alpha + 1)(c + \beta + 1)}{(c + 2)(c + \gamma + 1)} a_1 = \frac{(c + \alpha + 1)(c + \alpha)(c + \beta)(c + \beta + 1)}{(c + 2)(c + 1)(c + \gamma)(c + \gamma + 1)} a_0 = \frac{(c + \alpha)_2 (c + \beta)_2}{(c + 1)_2 (c + \gamma)_2} a_0 \\ a_3 &= \frac{(c + \alpha + 2)(c + \beta + 2)}{(c + 3)(c + \gamma + 2)} a_2 = \frac{(c + \alpha)_2 (c + \alpha + 2)(c + \beta )_2 (c + \beta + 2)}{(c + 1)_2 (c + 3)(c + \gamma)_2 (c + \gamma + 2)} a_0 = \frac{(c + \alpha)_3 (c + \beta)_3}{(c + 1)_3 (c + \gamma)_3} a_0 \end{align}

As we can see,

Hence, our assumed solution takes the form

We are now ready to study the solutions corresponding to the different cases for c1 − c2 = γ − 1 (this reduces to studying the nature of the parameter γ: whether it is an integer or not).

Read more about this topic:  Frobenius Solution To The Hypergeometric Equation

Famous quotes containing the word solution:

    “What is history? Its beginning is that of the centuries of systematic work devoted to the solution of the enigma of death, so that death itself may eventually be overcome. That is why people write symphonies, and why they discover mathematical infinity and electromagnetic waves.”
    —Boris Pasternak (1890–1960)