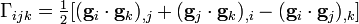Finite Strain Theory - Deformation Tensors in Curvilinear Coordinates | Deformation Tensors Curvilinear Coordinates

Deformation Tensors in Curvilinear Coordinates

A representation of deformation tensors in curvilinear coordinates is useful for many problems in continuum mechanics such as nonlinear shell theories and large plastic deformations. Let be a given deformation where the space is characterized by the coordinates . The tangent vector to the coordinate curve at is given by

$\mathbf{g}_i = \frac{\partial \mathbf{x}}{\partial \xi^i}$

The three tangent vectors at form a basis. These vectors are related the reciprocal basis vectors by

$\mathbf{g}_i\cdot\mathbf{g}^j = \delta_i^j$

Let us define a field

$g_{ij} := \frac{\partial \mathbf{x}}{\partial \xi^i}\cdot\frac{\partial \mathbf{x}}{\partial \xi^j} = \mathbf{g}_i\cdot\mathbf{g}_j$

The Christoffel symbols of the first kind can be expressed as

$\Gamma_{ijk} = \tfrac{1}{2}$

To see how the Christoffel symbols are related to the Right Cauchy-Green deformation tensor let us define two sets of bases

$\mathbf{G}_i := \frac{\partial \mathbf{X}}{\partial \xi^i} ~;~~ \mathbf{G}_i\cdot\mathbf{G}^j = \delta_i^j ~;~~ \mathbf{g}_i := \frac{\partial \mathbf{x}}{\partial \xi^i} ~;~~ \mathbf{g}_i\cdot\mathbf{g}^j = \delta_i^j$

Read more about this topic: Finite Strain Theory