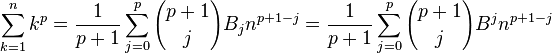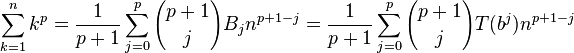Faulhaber's Formula - Umbral Form

Umbral Form

In the classic umbral calculus one formally treats the indices j in a sequence Bj as if they were exponents, so that, in this case we can apply the binomial theorem and say

\sum_{k=1}^n k^p = {1 \over p+1} \sum_{j=0}^p {p+1 \choose j} B_j n^{p+1-j} = {1 \over p+1} \sum_{j=0}^p {p+1 \choose j} B^j n^{p+1-j}


In the modern umbral calculus, one considers the linear functional T on the vector space of polynomials in a variable b given by

Then one can say

\sum_{k=1}^n k^p = {1 \over p+1} \sum_{j=0}^p {p+1 \choose j} B_j n^{p+1-j} = {1 \over p+1} \sum_{j=0}^p {p+1 \choose j} T(b^j) n^{p+1-j}


= {1 \over p+1} T\left(\sum_{j=0}^p {p+1 \choose j} b^j n^{p+1-j} \right) = T\left({(b+n)^{p+1} - b^{p+1} \over p+1}\right).

Read more about this topic:  Faulhaber's Formula

Famous quotes containing the word form:

    “The habits of life form the soul, and the soul forms the countenance.”
    —Honoré De Balzac (1799–1850)