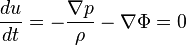Eddington Luminosity - Derivation

Derivation

The limit is obtained by setting the outward continuum radiation pressure equal to the inward gravitational force. Both forces decrease by inverse square laws, so once equality is reached, the hydrodynamic flow is different throughout the star.

From the Euler's equation in hydrostatic equilibrium, the mean acceleration is zero,

\frac{d u}{d t} = - \frac{\nabla p}{\rho} - \nabla \Phi = 0

where is the velocity, is the pressure, is the density, and is the gravitational potential. If the pressure is dominated by radiation associated to a radiation flux ,

-\frac{\nabla p}{\rho} = \frac{\kappa}{c} F_{\rm rad}\,.

Here is the opacity of the stellar material. For ionized hydrogen, where is the Thomson scattering cross-section for the electron and is the mass of a proton.

The luminosity of a source bounded by a surface is

L = \int_S F_{\rm rad} \cdot dS = \int_S \frac{c}{\kappa} \nabla \Phi \cdot dS\,.

Now assuming that the opacity is a constant, it can be brought outside of the integral. Using Gauss's theorem and Poisson's equation gives

L = \frac{c}{\kappa} \int_S \nabla \Phi \cdot dS = \frac{c}{\kappa} \int_V \nabla^2 \Phi \, dV = \frac{4 \pi G c}{\kappa} \int_V \rho \, dV = \frac{4 \pi G M c}{\kappa}

where is the mass of the central object. This is called the Eddington Luminosity. For pure ionized hydrogen

\begin{align}L_{\rm Edd}&=\frac{4\pi G M m_{\rm p} c} {\sigma_{\rm T}}\\ &\cong 1.26\times10^{31}\left(\frac{M}{M_\bigodot}\right){\rm W} = 3.2\times10^4\left(\frac{M}{M_\bigodot}\right) L_\bigodot \end{align}

where ☉ the mass of the Sun and ☉ the luminosity of the Sun.

The maximum luminosity of a source in hydrostatic equilibrium is the Eddington luminosity. If the luminosity exceeds the Eddington limit, then the radiation pressure drives an outflow. Note that contrary to the standard misconception, the Eddington limit does not require spherical symmetry. Indeed, this limit is utilized for nonspherical systems such as accretion disks.

The mass of the proton appears because, in the typical environment for the outer layers of a star, the radiation pressure acts on electrons, which are driven away from the center. Because protons are negligibly pressured by the analog of Thomson scattering, due to their larger mass, the result is to create a slight charge separation and therefore a radially directed electric field, acting to lift the positive charges, which are typically free protons under the conditions in stellar atmospheres. When the outward electric field is sufficient to levitate the protons against gravity, both electrons and protons are expelled together.

Read more about this topic:  Eddington Luminosity