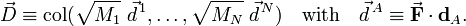Eckart Conditions - Separation of External and Internal Coordinates

Separation of External and Internal Coordinates

The N position vectors of the nuclei constitute a 3N dimensional linear space R3N: the configuration space. The Eckart conditions give an orthogonal direct sum decomposition of this space

$\mathbf{R}^{3N} = \mathbf{R}_\textrm{ext}\oplus\mathbf{R}_\textrm{int}.$

The elements of the 3N-6 dimensional subspace R_int are referred to as internal coordinates, because they are invariant under overall translation and rotation of the molecule and, thus, depend only on the internal (vibrational) motions. The elements of the 6-dimensional subspace R_ext are referred to as external coordinates, because they are associated with the overall translation and rotation of the molecule.

To clarify this nomenclature we define first a basis for R_ext. To that end we introduce the following 6 vectors (i=1,2,3):

$\begin{align} \vec{s}^A_{i} &\equiv \vec{f}_i \\ \vec{s}^A_{i+3} &\equiv \vec{f}_i \times\vec{R}_A^0 .\\ \end{align}$

An orthogonal, unnormalized, basis for R_ext is,

$\vec{S}_t \equiv \operatorname{row}(\sqrt{M_1}\;\vec{s}^{\,1}_{t}, \ldots, \sqrt{M_N} \;\vec{s}^{\,N}_{t}) \quad\mathrm{for}\quad t=1,\ldots, 6.$

A mass-weighted displacement vector can be written as

$\vec{D} \equiv \operatorname{col}(\sqrt{M_1}\;\vec{d}^{\,1}, \ldots, \sqrt{M_N}\;\vec{d}^{\,N}) \quad\mathrm{with}\quad \vec{d}^{\,A} \equiv \vec{\mathbf{F}}\cdot \mathbf{d}_A .$

For i=1,2,3,

$\vec{S}_i \cdot \vec{D} = \sum_{A=1}^N \; M_A \vec{s}^{\,A}_i \cdot \vec{d}^{\,A} =\sum_{A=1}^N M_A d_{Ai} = 0,$

where the zero follows because of the translational Eckart conditions. For i=4,5,6

$\, \vec{S}_i \cdot \vec{D} = \sum_{A=1}^N \; M_A \big(\vec{f}_i \times\vec{R}_A^0\big) \cdot \vec{d}^{\,A}=\vec{f}_i \cdot \sum_{A=1}^N M_A \vec{R}_A^0 \times\vec{d}^A = \sum_{A=1}^N M_A \big( \mathbf{R}_A^0 \times \mathbf{d}_A\big)_i = 0,$

where the zero follows because of the rotational Eckart conditions. We conclude that the displacement vector belongs to the orthogonal complement of R_ext, so that it is an internal vector.

We obtain a basis for the internal space by defining 3N-6 linearly independent vectors

$\vec{Q}_r \equiv \operatorname{row}(\frac{1}{\sqrt{M_1}}\;\vec{q}_r^{\,1}, \ldots, \frac{1}{\sqrt{M_N}}\;\vec{q}_r^{\,N}), \quad\mathrm{for}\quad r=1,\ldots, 3N-6.$

The vectors could be Wilson's s-vectors or could be obtained in the harmonic approximation by diagonalizing the Hessian of V. We next introduce internal (vibrational) modes,

$q_r \equiv \vec{Q}_r \cdot \vec{D} = \sum_{A=1}^N \vec{q}^A_r \cdot \vec{d}^{\,A} \quad\mathrm{for}\quad r=1,\ldots, 3N-6.$

The physical meaning of q_r depends on the vectors . For instance, q_r could be a symmetric stretching mode, in which two C—H bonds are simultaneously stretched and contracted.

We already saw that the corresponding external modes are zero because of the Eckart conditions,

$s_t \equiv \vec{S}_t \cdot \vec{D} = \sum_{A=1}^N M_A \;\vec{s}^{\,A}_t \cdot \vec{d}^{\,A} = 0 \quad\mathrm{for}\quad t=1,\ldots, 6.$

Read more about this topic: Eckart Conditions

Famous quotes containing the words separation, external and/or internal:

“Reunion after long separation is even better than one’s wedding night.”
—Chinese proverb.

“Many are poets but without the name,
For what is poesy but to create
From overfeeling good or ill; and aim
At an external life beyond our fate,
And be the new Prometheus of new men.”
—George Gordon Noel Byron (1788–1824)

“A State, in idea, is the opposite of a Church. A State regards classes, and not individuals; and it estimates classes, not by internal merit, but external accidents, as property, birth, etc. But a church does the reverse of this, and disregards all external accidents, and looks at men as individual persons, allowing no gradations of ranks, but such as greater or less wisdom, learning, and holiness ought to confer. A Church is, therefore, in idea, the only pure democracy.”
—Samuel Taylor Coleridge (1772–1834)