Daniell Integral - The Daniell Axioms

The Daniell Axioms

We start by choosing a family of bounded real functions (called elementary functions) defined over some set, that satisfies these two axioms:

1. is a linear space with the usual operations of addition and scalar multiplication.

2. If a function is in, so is its absolute value .

In addition, every function h in H is assigned a real number, which is called the elementary integral of h, satisfying these three axioms:

1. Linearity. If h and k are both in H, and and are any two real numbers, then .

2. Nonnegativity. If, then .

3. Continuity. If is a nonincreasing sequence (i.e. ) of functions in that converges to 0 for all in, then .

That is, we define a continuous non-negative linear functional over the space of elementary functions.

These elementary functions and their elementary integrals may be any set of functions and definitions of integrals over these functions which satisfy these axioms. The family of all step functions evidently satisfies the above axioms for elementary functions. Defining the elementary integral of the family of step functions as the (signed) area underneath a step function evidently satisfies the given axioms for an elementary integral. Applying the construction of the Daniell integral described further below using step functions as elementary functions produces a definition of an integral equivalent to the Lebesgue integral. Using the family of all continuous functions as the elementary functions and the traditional Riemann integral as the elementary integral is also possible, however, this will yield an integral that is also equivalent to Lebesgue's definition. Doing the same, but using the Riemann–Stieltjes integral, along with an appropriate function of bounded variation, gives a definition of integral equivalent to the Lebesgue–Stieltjes integral.

Sets of measure zero may be defined in terms of elementary functions as follows. A set which is a subset of is a set of measure zero if for any, there exists a nondecreasing sequence of nonnegative elementary functions in H such that and 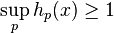on .

A set is called a set of full measure if its complement, relative to, is a set of measure zero. We say that if some property holds at every point of a set of full measure (or equivalently everywhere except on a set of measure zero), it holds almost everywhere.