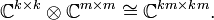Choi's Theorem On Completely Positive Maps - Some Preliminary Notions

Some Preliminary Notions

Before stating Choi's result, we give the definition of a completely positive map and fix some notation. Cn × n will denote the C*-algebra of n × n complex matrices. We will call A ∈ Cn × n positive, or symbolically, A ≥ 0, if A is Hermitian and the spectrum of A is nonnegative. (This condition is also called positive semidefinite.)

A linear map Φ : Cn × n → Cm × m is said to be a positive map if Φ(A) ≥ 0 for all A ≥ 0. In other words, a map Φ is positive if it preserves Hermiticity and the cone of positive elements.

Any linear map Φ induces another map

in a natural way: define

( I_k \otimes \Phi ) (M \otimes A) = M \otimes \Phi (A)

and extend by linearity. In matrix notation, a general element in

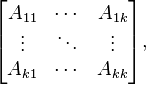
can be expressed as a k × k operator matrix:

\begin{bmatrix} A_{11} & \cdots & A_{1k} \\ \vdots & \ddots & \vdots \\ A_{k1} & \cdots & A_{kk} \end{bmatrix},

and its image under the induced map is

(I_k \otimes \Phi) (\begin{bmatrix} A_{11} & \cdots & A_{1k} \\ \vdots & \ddots & \vdots \\A_{k1} & \cdots & A_{kk} \end{bmatrix}) = \begin{bmatrix} \Phi (A_{11}) & \cdots & \Phi( A_{1k} ) \\ \vdots & \ddots & \vdots \\ \Phi (A_{k1}) & \cdots & \Phi( A_{kk} ) \end{bmatrix}.

Writing out the individual elements in the above matrix-of-matrices amounts to the natural identification of algebras

\mathbb{C}^{k\times k}\otimes\mathbb{C}^{m\times m}\cong\mathbb{C}^{km\times km}.

We say that Φ is k-positive if, considered as an element of Ckm×km, is a positive map, and Φ is called completely positive if Φ is k-positive for all k.

The transposition map is a standard example of a positive map that fails to be 2-positive. Let T denote this map on C 2 × 2. The following is a positive matrix in :

\begin{bmatrix} \begin{pmatrix}1&0\\0&0\end{pmatrix}& \begin{pmatrix}0&1\\0&0\end{pmatrix}\\ \begin{pmatrix}0&0\\1&0\end{pmatrix}& \begin{pmatrix}0&0\\0&1\end{pmatrix} \end{bmatrix} = \begin{bmatrix} 1 & 0 & 0 & 1 \\ 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 \\ 1 & 0 & 0 & 1 \\ \end{bmatrix} .

The image of this matrix under is

\begin{bmatrix} \begin{pmatrix}1&0\\0&0\end{pmatrix}^T& \begin{pmatrix}0&1\\0&0\end{pmatrix}^T\\ \begin{pmatrix}0&0\\1&0\end{pmatrix}^T& \begin{pmatrix}0&0\\0&1\end{pmatrix}^T \end{bmatrix} = \begin{bmatrix} 1 & 0 & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 1 \\ \end{bmatrix} ,

which is clearly not positive, having determinant -1.

Incidentally, a map Φ is said to be co-positive if the composition Φ T is positive. The transposition map itself is a co-positive map.

The above notions concerning positive maps extend naturally to maps between C*-algebras.

Read more about this topic:  Choi's Theorem On Completely Positive Maps

Famous quotes containing the words preliminary and/or notions:

    “Religion is the state of being grasped by an ultimate concern, a concern which qualifies all other concerns as preliminary and which itself contains the answer to the question of a meaning of our life.”
    —Paul Tillich (1886–1965)

    “Even the simple act that we call “going to visit a person of our acquaintance” is in part an intellectual act. We fill the physical appearance of the person we see with all the notions we have about him, and in the totality of our impressions about him, these notions play the most important role.”
    —Marcel Proust (1871–1922)